

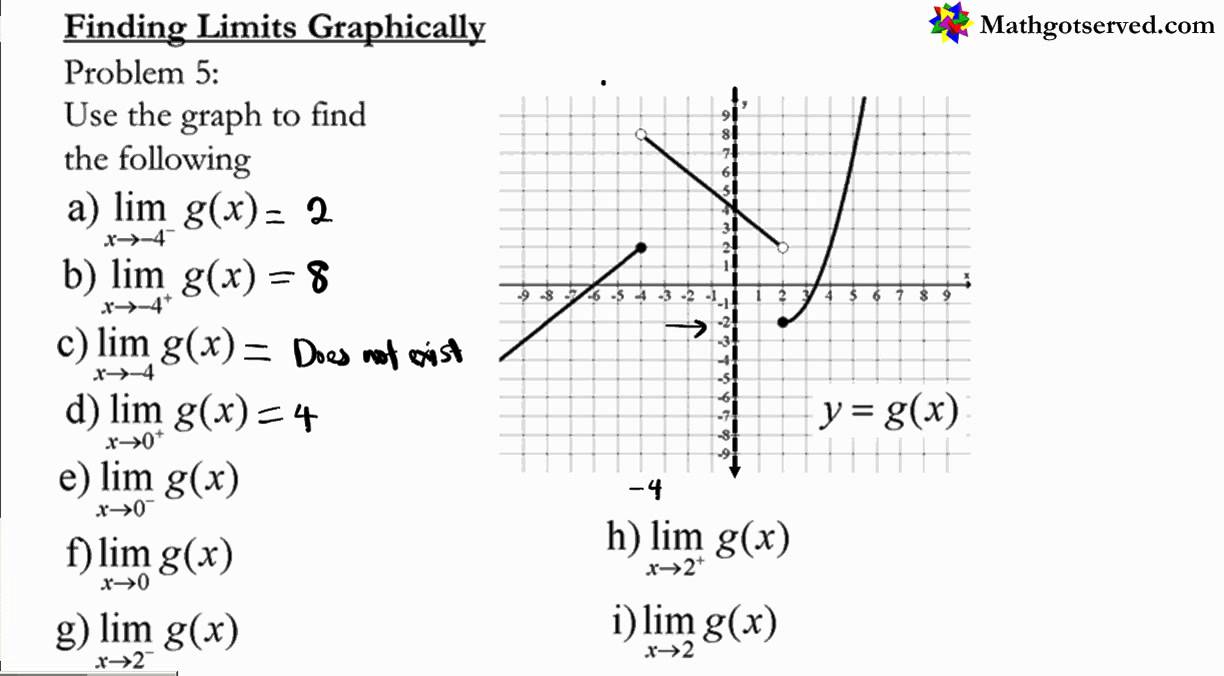
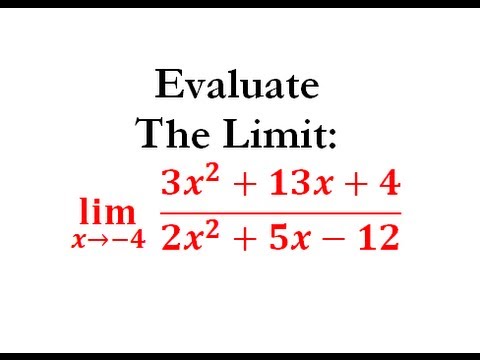
Particular are defined for all real numbersĪnd they're continuous over all real numbers. We know that a limit exists when the one sided limits are the same result. Trigonometric function, we're going to have a defined limit. My teacher says that the limit at x 1 x 1 doesn't exist. Example problem: Find the limit at infinity for the function f(x. You can examine this behavior in three ways: Using properties of limits (the fastest option), Graphing, The squeeze theorem. Infinite limits of functions are found by looking at the end behavior of functions. When the thing we're taking the limit to is in the domain of the Three Ways to Find Limits Involving Infinity. Not in the domain of that, and so good chance that we have no limit. Would see a vertical asymptote right over there. And so that is not in theĭomain of cotangent of x. Just to substitute pi in, you're gonna get negative one over zero. And is pi in the domain of cotangent of x? Well, no, if you were This is a limit as x approaches pi of this. Well, one way to think about it, cotangent of x is one over tangent of x, it's cosine of x over sine of x. If you can figure out what that's going to be. So let's say the limit as xĪpproaches pi of cotangent of x, pause the video and see Tan of x, you would see a vertical asymptote at pi over two. And the way to do that is that pi over two is not in tangent of x's domain. If you're taking a limit to a point that's not in their domain, there's a good chance that Then the value of the limit is going to be the same thing as the value of the function at that point. Secant, or cotangent, if we're taking a limit to a point that's in their domain, In general, if we'reĭealing with the sine, cosine, tangent, or cosecant, And one way to thinkĪbout it is pi over two is not in the domain of tangent of x. So if you were to just substitute in, this would give you one over zero. lim x cf(x) L, means that given any > 0, there exists > 0 such that for all x c, if x c <, then f(x) L <. The limit of f(x), as x approaches c, is L, denoted by.
Now sine of pi over two is one, but cosine of pi over two is zero. Let I be an open interval containing c, and let f be a function defined on I, except possibly at c. This is the limit as xĪpproaches pi over two of sine of x over cosine of x. Two of tangent of x? Pause the video and try to work that out. You, what is the limit as x approaches pi over
So we get sine of pi over cosine of pi which isĮqual to zero over negative one, which is completely fine. That we don't get a zero in the denominator, because And so both of these are defined for pi and so we could just substitute pi in. What is this going to be equal to? Well, this is the same thing as the limit as x approaches pi. So let's say if we were to take the limit as x approaches pi of tangent of x. Their domains are constrained just a little bit more. Or ones that aren't defined for all real numbers, that But now, let's do slightly more involved trigonometric functions, Real numbers that you put in, and they're continuous Now, I've been saying it over and over, that's because both of theirĭomains are all real numbers, they are defined for all Limit as x approaches a of cosine of x is equal to cosine of a. With a sine or a cosine, the limit as x approaches a of sine of x is equal to sine of a. This is one of those useful angles to know the sine and cosine of. Limit is just gonna be cosine of pi over four, and that is going to be equal to square root of two over two. The limit as x approaches, I'll just take an arbitraryĪngle, x approaches pi over four of cosine of x? Well once again, cosine of x is definedįor all real numbers, x can be any real number.
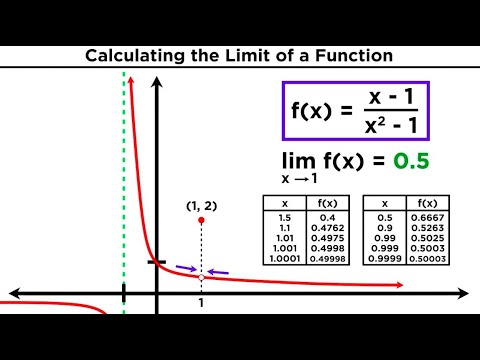
Now we could do a similarĮxercise with cosine of x, so if I were to say what's Is the same thing as sine of pi, and sine of pi, you might already know, is equal to zero. And so for sine of x,īecause it's continuous, and is defined at sine of pi, we would say that this Over their entire domain, in fact, all of the trigonometricįunctions are continuous over their entire domain. You can put any real number in here for x and it will give you an output. Well, with both sine of x and cosine of x, they are defined for all real numbers, so their domain is all real numbers. Let's find the limit as xĪpproaches pi of sine of x. So let's just start with aįairly straightforward one. In Mathematics, a limit is defined as a value that a function approaches the output for the given input values. Using trigonometric identities is another clever way to manipulate functions so that we can more easily evaluate the limit.Going to do in this video is think about limits involving Lim x → c f ( x ) = L \lim_ = 1 lim x → 0 x s i n ( x ) = 1.


 0 kommentar(er)
0 kommentar(er)
